7 Division Concepts
Like multiplication, division concepts are built on subtraction and skip counting principles. Often we refer to division as “repeated subtraction.” However, division is more than this simple phrase implies.
Learners need to understand that division is separating or taking apart (like in subtraction) “groups of equal size.” Division is the act of decomposing a number into its factors. The factors represent the size of each group and the number of groups.
EXPLORING DIVISION CONCEPTS
- Have learners show a number on the Nudget. Ensure the number is not prime.
- Guide learners in counting backward by groups of size 3.
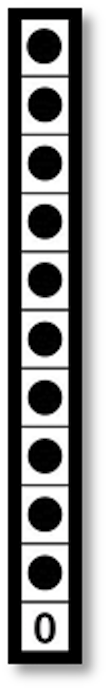
Start with the number 9.
Skip count backwards by 3.
Use tally marks to keep track.
Count, “1 group.” 
 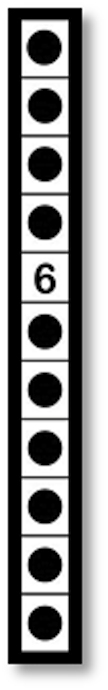 |
Skip count backwards by 3.
Count, “2 groups.” 
 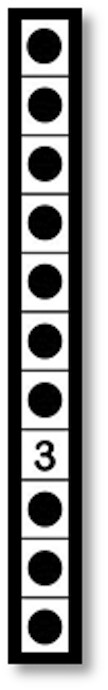 |
Skip count backwards by 3.
Count, “3 groups.” 
  |
Thus, 9 is decomposed into 3 groups of 3 checkers.
Learners should understand that fully decomposing a number results in 0. Facilitate learners in recognizing that each slide is a group and the number of checkers moved represents the size of the group. Learners should gain fluency in sliding equal numbers of checkers and counting the groups to ensure this concept is fully developed.
BASIC DIVISION FACTS
- Have learners count backwards by groups of size 4, writing the number of groups counted.
2. Guide learners in recognizing that they are taking apart a number by groups of size 4 to fully decompose the number.
Start with the number 20 and skip count backwards by 4.
The Nudget must be regrouped for counting to begin.
Counting back 1 group of 4 results in 16.
Counting back 2 groups of 4 results in 12.
 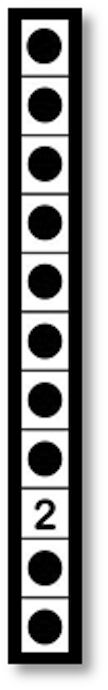 |
Counting back 3 groups of 4 requires regrouping to continue counting the group.
The result is 8.
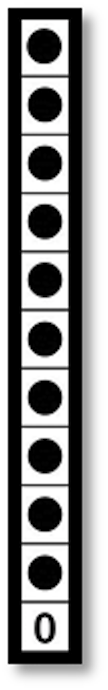 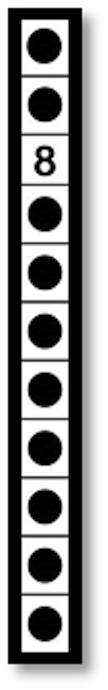 |
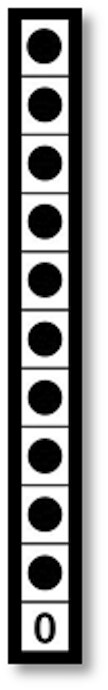
Counting back 4 groups of 4 results in 4.
 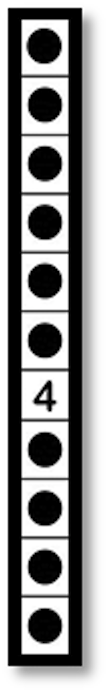 |
Counting back 5 groups of 4 results in 0.
 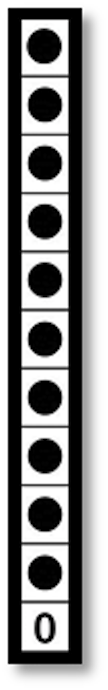 |
Write: 20 ÷ 4 = 5
3. Have learners decompose the numbers 16, 12, and 8 by groups of size 4, writing the number of groups counted.
The division fact family of 4:
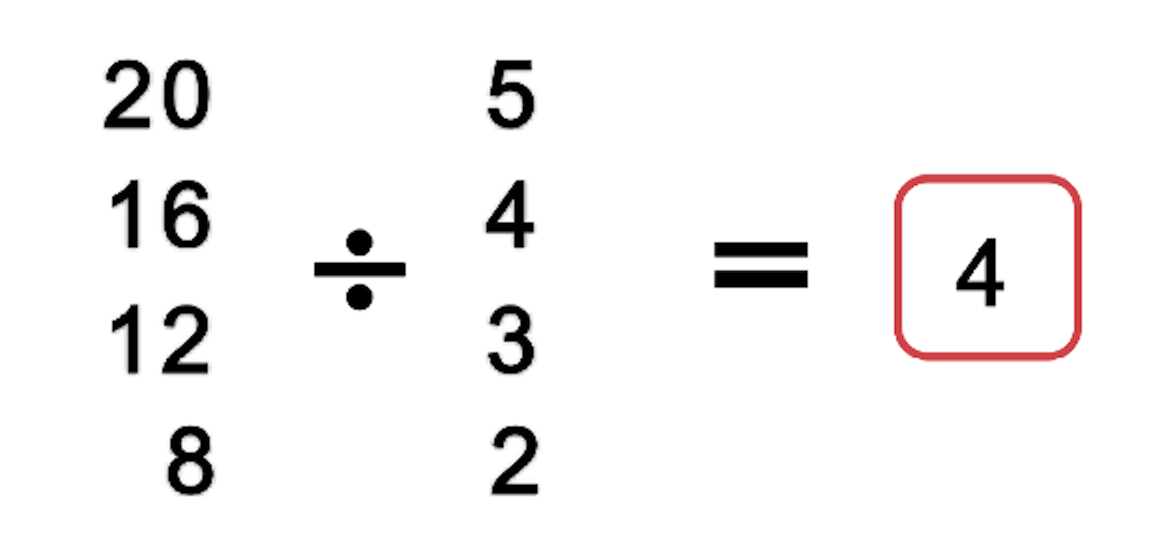
Dividend Groups Size
Provide learners opportunity to master fact families through groups of size 12. Facilitate learners in connecting the dividend separated into groups of a size principle with multiplication. It is important that fluency of division facts is developed as well as understanding the relationship between multiplication and division facts.
DEVELOPING REMAINDER CONCEPTS
1. Have learners hypothesize about what would happen if you could not fully decompose a number.
2. Have learners show 23 on the Nudget and decompose the number by groups of 5.
Start with the number 23 and skip count backwards by 5.
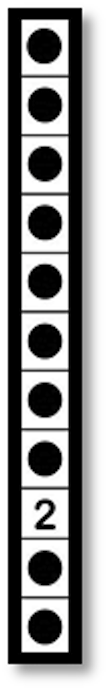 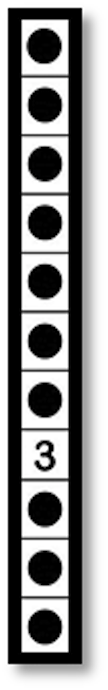 |
Only 3 checkers are counted before a 10 is regrouped to continue counting 1 group of 5.
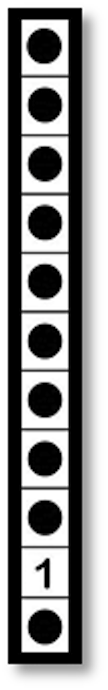 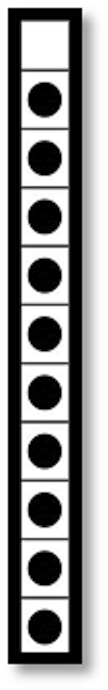 |
Counting back 2 more checkers.
1 group of 5 results in 18.
 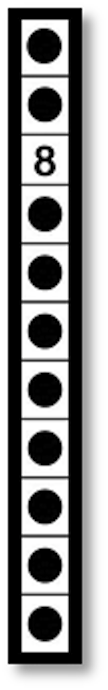 |
Counting back 2 groups of 5 results in 13.
 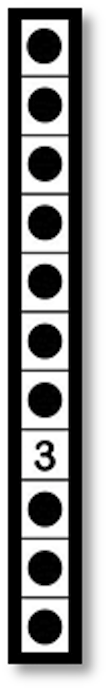 |
Counting back 3 groups of 5 requires regrouping to continue counting the group. The results is 8.
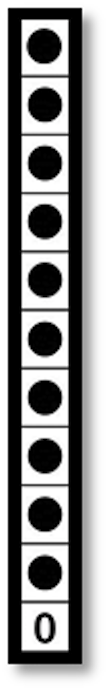 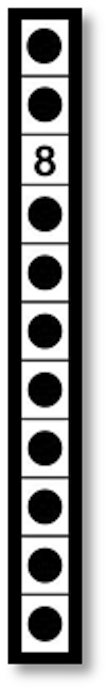 |
Counting back 4 groups of 5 results in 3.
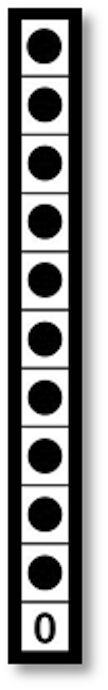 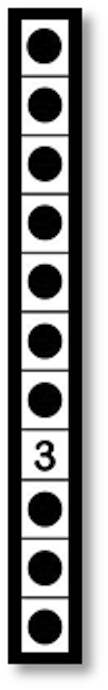 |
Write: 23 ÷ 5 = 4 R 3
Thus, 23 is divided into 4 groups of size 5, with 3 checkers remaining.
Provide learners opportunity to decompose numbers that result in remainders. Encourage learners to find efficient strategies for finding remainders quickly. Learners should develop the conceptual understanding of remainders prior to working with traditional algorithms or fractional remainders.